Introduction to Graph Theory

Introduction to Graph Theory
HANDBOOK OF GRAPH THEORY FOR FRESHER'S

Prem Sankar C
M Tech Technology Management
Dept of Futures Studies ,Kerala University

Outline
1.
2.
3.
4.
5.

History of Graph Theory
Basic Concepts of Graph Theory
Graph Representations
Graph Terminologies
Different Type of Graphs

Why Graph Theory ?

 Graphs used to model pair wise relations between

objects
 Generally a network can be represented by a graph
 Many practical problems can be easily represented
in terms of graph theory

Graph Theory - History
The origin of graph theory can be traced back to Euler's work on the
Konigsberg bridges problem (1735), which led to the concept of an
Eulerian graph. The study of cycles on polyhedra by the Thomas P.
Kirkman (1806 - 95) and William R. Hamilton (1805-65) led to the
concept of a Hamiltonian graph.

Graph Theory - History
 Begun in 1735
 Mentioned in Leonhard Euler's

paper on “Seven Bridges of
Konigsberg ” .

Problem : Walk all 7 bridges
without crossing a bridge twice

Graph Theory – History…….
Cycles in Polyhedra - polyhedron with no Hamiltonian cycle

Thomas P. Kirkman

William R. Hamilton

Hamiltonian cycles in Platonic graphs

Graph Theory – History…..
Trees in Electric Circuits

Gustav Kirchhoff

Basic Concepts of Graph Theory

Definition: Graph
 A graph is a collection of nodes and edges

 Denoted by G = (V, E).

V = nodes (vertices, points).
E = edges (links, arcs) between pairs of nodes.
Graph size parameters: n = |V|, m = |E|.


Vertex & Edge
 Vertex /Node





Basic Element
Drawn as a node or a dot.
Vertex set of G is usually denoted by V(G), or V or VG

 Edge /Arcs




A set of two elements
Drawn as a line connecting two vertices, called end vertices, or
endpoints.
The edge set of G is usually denoted by E(G), or E or EG

 Neighborhood


For any node v, the set of nodes it is connected to via an edge is
called its neighborhood and is represented as N(v)

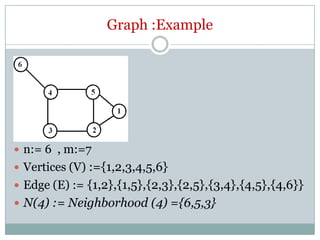
Graph :Example

 n:= 6 , m:=7
 Vertices (V) :={1,2,3,4,5,6}

{1,2},{1,5},{2,3},{2,5},{3,4},{4,5},{4,6}}
 N(4) := Neighborhood (4) ={6,5,3}
 Edge (E) :=

Edge types:


Undirected;




Directed; ordered pairs of nodes.





E.g., distance between two cities, friendships…
E.g ,…
Directed edges have a source (head, origin) and target (tail,
destination) vertices

Weighted ; usually weight is associated .

Empty Graph / Edgeless graph
 No edge

 Null graph
 No nodes
 Obviously no edge

Simple Graph (Undirected)
 Simple Graph are undirected graphs without loop or

multiple edges
 A = AT

F or sim ple graphs,

deg( v i )
vi V

2|E |

Directed graph : (digraph)
 Edges have directions
 A !=AT

loop
multiple arc

arc

node

Weighted graph
 is a graph for which each edge has an associated weight

1

2

1.2
2

3

.2
.3

.5
4

1.5
5

.5

1

6

5

1

4

3

2

5

3
6

Bipartite Graph
V can be partitioned into 2 sets V1 and V2
such that (u,v) E implies
either u V1 and v V2
OR v V1 and u V2.

Trees
 An undirected graph is a tree if it is connected and does not

contain a cycle (Connected Acyclic Graph)
 Two nodes have exactly one path between them

Subgraph
 Vertex and edge sets are subsets of those of G
 a supergraph of a graph G is a graph that contains G as a
subgraph.

1. Adjacency Matrix
 n-by-n matrix with Auv = 1 if (u, v) is an edge.



Diagonal Entries are self-links or loops
Symmetric matrix for undirected graphs

1
2
3
4
5
6
7
8

1
0
1
1
0
0
0
0
0

2
1
0
1
1
1
0
0
0

3
1
1
0
0
1
0
1
1

4
0
1
0
1
1
0
0
0

5
0
1
1
1
0
1
0
0

6
0
0
0
0
1
0
0
0

7
0
0
1
0
0
0
0
1

8
0
0
1
0
0
0
1
0

2. Incidence Matrix



VxE
[vertex, edges] contains the edge's data

1, 2

1,5

2 ,3

2 ,5

3, 4

4 ,5

4 ,6

1

1

1

0

0

0

0

0

2

1

0

1

1

0

0

0

3

0

0

1

0

1

0

0

4

0

0

0

0

1

1

1

5

0

1

0

1

0

1

0

6

0

0

0

0

0

0

1

3. Adjacency List
 Edge List

Edge List
12
12
23
25
33
43
45
53
54

 Adjacency List (node list)

Node List
122
235
33
435
534

Edge Lists for Weighted Graphs

Edge List
1 2 1.2
2 4 0.2
4 5 0.3
4 1 0.5
5 4 0.5
6 3 1.5

Classification of Graph Terms



Global terms refer to a whole graph
Local terms refer to a single node in a graph

Connected and Isolated vertex

 Two vertices are connected if there is a path

between them
 Isolated vertex – not connected
1

isolated vertex

2

3

4

5

6

Adjacent nodes
 Adjacent nodes -Two nodes are adjacent if they

are connected via an edge.
 If edge e={u,v} ∈ E(G), we say that u and v are adjacent or neigbors

 An edge where the two end vertices are the same is called a

loop, or a self-loop

Degree (Un Directed Graphs)
 Number of edges incident on a node

The degree of 5 is 3

Degree (Directed Graphs)


In-degree: Number of edges entering



Out-degree: Number of edges leaving



Degree = indeg + outdeg

outdeg(1)=2
indeg(1)=0
outdeg(2)=2
indeg(2)=2
outdeg(3)=1
indeg(3)=4

Walk
 trail: no edge can be repeat

a-b-c-d-e-b-d

 walk: a

path in which edges/nodes
can be repeated.
a-b-d-a-b-c

 A walk is closed is a=c

Paths
 Path: is a sequence P of nodes v1, v2, …, vk-1, vk
 No vertex can be repeated

 A closed path is called a cycle
 The length of a path or cycle is the number of edges visited in the path

or cycle

1,2,5,2,3,4
walk of length 5

Walks and Paths
1,2,5,2,3,2,1
CW of length 6

1,2,3,4,6
path of length 4

Cycle
 Cycle - closed path: cycle (a-b-c-d-a) , closed if x=y
 Cycles denoted by Ck, where k is the number of nodes in the

cycle

C3

C4

C5

Shortest Path
 Shortest Path is the path between two nodes

that has the shortest length
 Length – number of edges.
 Distance between u and v is the length of a shortest
path between them
 The diameter of a graph is the length of the longest
shortest path between any pairs of nodes in the
graph

THANK YOU
Prem Sankar C
M Tech Technology Management
Dept of Futures Studies
Kerala University

Prem Sankar C - Dept of Futures Studies

Introduction to Graph Theory

Recommended

More Related Content

What's hot (20)

Viewers also liked (19)

Similar to Introduction to Graph Theory (20)

More from Premsankar Chakkingal (13)

Recently uploaded (20)

Introduction to Graph Theory