Lecture 13 (Usage of Fourier transform in image processing)

Oct 8, 20200 likes810 views

This document discusses the Fourier transform and its applications in image processing. It begins by explaining the Fourier transform for 1D and 2D continuous and discrete signals. The Fourier transform converts a signal from the time or space domain to the frequency domain. It then covers properties of the Fourier transform such as separability and translation. The document concludes by mentioning references for further reading on image processing and computer vision topics.

Usage of Fourier Transform in Image Processing
Subject: Image Procesing & Computer Vision
Dr. Varun Kumar
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 1 / 13

Outlines
1 Fourier transform for continuous signal
2 Fourier transform for discrete signal
3 Fast Fourier transform
4 References
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 2 / 13

Fourier transform of 1D time varying signal
It is a mathematical operation that convert time domain signal to
frequency domain signal.
Frequency domain signal processing is simpler compare to time
domain.
Mathematical expression:
1 Fourier transform
X(jΩ) =
∞
−∞
x(t)e−jΩt
dt
CTFT
X(ejω
) =
∞
−∞
x(n)e−jωn
DTFT
(1)
2 Inverse Fourier transform
x(t) =
1
2π
∞
−∞
X(jΩ)ejΩt
dΩ
I−CTFT
x(n) =
1
2π
∞
−∞
X(ejω
)ejωn
I−DTFT
(2)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 3 / 13

Fourier transform of 1D space varying signal
1 Fourier transform
F(f (x)) = F(u) =
∞
−∞
f (x)e−j2πux
dx (3)
⇒ Here, f (x) must be continuous and integrable.
⇒ F(u) must be integrable.
2 Inverse Fourier transform
F−1
(F(u)) = f (x) =
∞
−∞
F(u)ej2πux
du (4)
⇒ F(u) is a complex variable.
⇒ F(u) = FRe(u) + jFIm(u) = |F(u)|ejφ(u)
⇒ Amplitude spectrum : |F(u)| = FRe(u)2 + FIm(u)2
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 4 / 13

Fourier transform of 2D signal
⇒ Phase spectrum : ∠φ(u) = tan−1 FIm(u)
FRe (u)
⇒ Power: P = |F(u)|2 = FRe(u)|2 + |FIm(u)|2
Fourier transform of 2D signal
Image is a 2D space varying signal.
Fourier transform of an image signal
F(u, v) =
∞
−∞
∞
−∞
f (x, y)e−j2π(ux+vy)
dxdy (5)
where f (x, y) is the 2D image signal.
Inverse Fourier transform :
f (x, y) =
∞
−∞
∞
−∞
F(u, v)ej2π(ux+vy)
dudv (6)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 5 / 13

Continued–
⇒ F(u, v) = |F(u, v)|ejφ(u,v)
⇒ Amplitude spectrum : |F(u, v)| = FRe(u, v)2 + FIm(u, v)2
⇒ Phase spectrum : φ(u, v) = tan−1 FIm(u,v)
FRe (u,v)
⇒ Power spectrum : P(u, v) = |F(u, v)|2 = FRe(u, v)2 + FIm(u, v)2
Example : Find the Fourier transform of a 2D signal which is as follow
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 6 / 13

Continued–
As per the 2D graphical signal f (x, y)
⇒ f (x, y) = A ∀ 0 ≤ x ≤ X and 0 ≤ y ≤ Y
⇒ f (x, y) = 0 ∀ x > X and y > Y
F(u, v) =
X
0
Y
0
Ae−j(ux+vy)
dxdy
= A
X
0
e−j2πux
dx
Y
0
e−j2πvy
dy
= A
1
j2πu
(1 − e−j2πuX
)
1
j2πv
(1 − e−j2πvY
)
(7)
Amplitude spectrum :
|F(u, v)| = AXY
sin(πuX)
πuX
sin(πvY )
πvY
(8)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 7 / 13

Graphical representation
2D discrete signal (DTFT) (Finite dimension)
F(u, v) =
∞
−∞
∞
−∞
f (x, y)e−j2π(ux+vy)
(9)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 8 / 13

DFT of an image signal
DFT/I-DFT of an 2D signal
DFT
F(u, v) =
1
MN
M−1
x=0
N−1
y=0
f (x, y)e−j2π( ux
M
+vy
N
)
(10)
Here, frequency variable u = 0, 1, ....., M − 1 and v = 0, 1, ...., N − 1
I-DFT
f (x, y) =
1
MN
M−1
u=0
N−1
v=0
F(u, v)ej2π( ux
M
+vy
N
)
(11)
In case of square image, i.e, M = N
F(u, v) =
1
N
N−1
x=0
N−1
y=0
f (x, y)e−j2π( ux
N
+vy
N
)
(12)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 9 / 13

Properties of Fourier transform
1 Separability:
In case of DFT
F(u, v) =
1
N
N−1
x=0
N−1
y=0
f (x, y)e−j2π(ux
N
+vy
N
)
⇒
1
N
N−1
x=0
e−j 2πux
N N.
1
N
N−1
y=0
f (x, y)e−j 2πvy
N
⇒
1
N
N−1
x=0
NF(x, v)e−j 2πux
N
(13)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 10 / 13

Continued–
In case of I-DFT
f (x, y) =
1
N
N−1
u=0
N−1
v=0
F(u, v)ej2π( ux
N
+vy
N
)
⇒
1
N
N−1
u=0
ej 2πux
N N.
1
N
N−1
v=0
F(u, v)ej 2πvy
N
⇒
1
N
N−1
u=0
Nf (u, y)ej 2πux
N
(14)
2 Translation
f (x, y) ⇒ (x0, y0) ⇒ f (x − x0, y − y0)
F(u, v)|x−x0,y−y0 = F(u, v)|x,y e
−j2π
N
(ux0+vy0)
(15)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 11 / 13

Continued–
I-DFT form
F(u − u0, v − v0) ⇒ f (x, y)e
j2π
N
(u0x+v0y)
(16)
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 12 / 13

References
M. Sonka, V. Hlavac, and R. Boyle, Image processing, analysis, and machine vision.
Cengage Learning, 2014.
D. A. Forsyth and J. Ponce, “A modern approach,” Computer vision: a modern
approach, vol. 17, pp. 21–48, 2003.
L. Shapiro and G. Stockman, “Computer vision prentice hall,” Inc., New Jersey,
2001.
R. C. Gonzalez, R. E. Woods, and S. L. Eddins, Digital image processing using
MATLAB. Pearson Education India, 2004.
Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 13 / 13

This document discusses properties of the discrete Fourier transform (DFT) for 2D signals. It outlines properties including periodicity, conjugation, rotation, distributivity, scaling, and convolution/correlation. It also discusses how the fast Fourier transform (FFT) reduces the computational complexity of the DFT from O(N^4) to O(N^2logN) for 2D signals with N samples. The properties of the DFT allow operations in the frequency domain to correspond to operations in the spatial domain, which makes the Fourier transform useful for applications like image processing and computer vision.

Fourier transformsIffat Anjum

ppt on sustainable buildingsnavyavani kola

Graduation Project - PV Solar With MPPT SystemAhmed Nabil

Fuzzy logicBabu Appat

SAMPLING & RECONSTRUCTION OF DISCRETE TIME SIGNALkaran sati

Discrete time signals can be obtained by sampling an analog signal at regular intervals or by observing an inherently discrete process. Sampling is the process of breaking a continuous signal into discrete samples by recording the signal's value at time intervals called the sampling period. According to the sampling theorem, a signal can be uniquely reconstructed from its samples if it is sampled at a rate greater than twice its highest frequency component. Reconstruction involves isolating the baseband spectrum from the spectral images caused by sampling through the use of a low-pass filter, which corresponds to convolving the samples with a sinc function. Practical reconstruction uses an approximation to the ideal sinc filter.

JAVA PPT Part-1 BY ADI.pdfProf. Dr. K. Adisesha

The document discusses Java programming and provides details about: 1. Java is an object-oriented programming language that is platform independent and allows code to run on any system that supports Java. 2. The document covers Java history, features, platforms, data types, variables, operators, control structures and provides examples of Java programs. 3. Key concepts discussed include classes, objects, inheritance, polymorphism, encapsulation and abstraction in Java.

Lecture 16 KL Transform in Image ProcessingVARUN KUMAR

The KL transform is a data-driven transformation where the kernel is derived from the statistics of the data, unlike transforms like DFT where the kernel is fixed. (1) It represents data as a vector based on the mean and covariance matrix of the population. (2) The transformation matrix is chosen such that the transformed data is statistically uncorrelated and ordered by decreasing variance. (3) This transformation optimally compacts the energy but requires high computational complexity.

Chapter 5 Image Processing: Fourier TransformationVarun Ojha

This document discusses the Fourier transformation, including: 1) It defines continuous and discrete Fourier transformations and their properties such as separability, translation, periodicity, and convolution. 2) The fast Fourier transformation (FFT) improves the computational complexity of the discrete Fourier transformation from O(N^2) to O(NlogN). 3) FFT works by rewriting the DFT calculation in a way that exploits symmetry and reduces redundant computations.

Frequency Domain Image Enhancement TechniquesDiwaker Pant

The document discusses various techniques for enhancing digital images, including spatial domain and frequency domain methods. It describes how frequency domain techniques work by applying filters to the Fourier transform of an image, such as low-pass filters to smooth an image or high-pass filters to sharpen it. Specific filters discussed include ideal, Butterworth, and Gaussian filters. The document provides examples of applying low-pass and high-pass filters to images in the frequency domain.

Image Restoration (Digital Image Processing)Kalyan Acharjya

Smoothing Filters in Spatial DomainMadhu Bala

Unit iiChetan Selukar

The document discusses various image transforms. It begins by explaining why transforms are used, such as for fast computation and obtaining conceptual insights. It then introduces image transforms as unitary matrices that represent images using a discrete set of basis images. It proceeds to describe one-dimensional orthogonal and unitary transforms using matrices. It also discusses separable two-dimensional transforms and provides properties of unitary transforms such as energy conservation. Specific transforms discussed in more detail include the discrete Fourier transform, discrete cosine transform, discrete sine transform, and Hadamard transform.

06 spatial filtering DIPbabak danyal

Spatial filtering involves applying filters or kernels to images to enhance or modify pixel values based on neighboring pixel values. Linear spatial filtering involves taking a weighted sum of pixel values within the filter window. Common filters include averaging filters for noise reduction, median filters to reduce impulse noise while preserving edges, and sharpening filters like Laplacian filters and unsharp masking to enhance details.

Image sampling and quantizationBCET, Balasore

The document discusses image sampling and quantization. It defines a digital image as a discrete 2D array containing intensity values of finite bits. A digital image is formed by sampling a continuous image, which involves multiplying it by a comb function of discrete delta pulses, yielding discrete image values. Quantization further discretizes the intensity values into a finite set of values. For accurate image reconstruction, the sampling frequency must be greater than twice the maximum image frequency, as stated by the sampling theorem.

Wiener FilterAkshat Ratanpal

The Wiener filter is a signal processing filter that reduces noise in a signal. It was proposed by Norbert Wiener in 1940 and published in 1949. The Wiener filter takes a statistical approach to minimize the mean square error between an original noiseless signal and the estimated signal by assuming knowledge of the spectral properties of the original signal and noise. It is commonly used for noise reduction and image deblurring. The Wiener filter implementation is available in Matlab and Python and its performance depends on the noise parameters used.

Image Filtering in the Frequency DomainAmnaakhaan

Fourier transformNaveen Sihag

The Fourier transform decomposes a signal into its constituent frequencies, representing it in the frequency domain rather than the spatial domain, which can make certain operations and analyses easier to perform; it has both magnitude and phase components that provide information about the frequency content and relative phases of the signal. The discrete Fourier transform (DFT) is a sampled version of the continuous Fourier transform that is useful for digital signal and image processing applications.

Image Enhancement - Point ProcessingGayathri31093

Homomorphic filteringGautam Saxena

Homomorphic filtering is a technique used to remove multiplicative noise from images by transforming the image into the logarithmic domain, where the multiplicative components become additive. This allows the use of linear filters to separate the illumination and reflectance components, with a high-pass filter used to remove low-frequency illumination variations while preserving high-frequency reflectance edges. The filtered image is then transformed back to restore the original domain. Homomorphic filtering is commonly used to correct non-uniform illumination and simultaneously enhance contrast in grayscale images.

Histogram ProcessingAmnaakhaan

Chapter 1 and 2 gonzalez and woodsasodariyabhavesh

This document provides an overview of digital image processing and human vision. It discusses the key stages of digital image processing including image acquisition, enhancement, restoration, morphological processing, segmentation, representation and description, object recognition, and compression. It also covers the anatomy of the human eye, photoreceptors, color perception, image formation in the eye, brightness adaptation, and the Weber ratio relating the just noticeable difference in light intensity to background intensity. The document uses images and diagrams from the textbook "Digital Image Processing" to illustrate concepts in digital images and the human visual system.

Sharpening spatial filtersResearch Scholar in Manonmaniam Sundaranar University

its very useful for students. Sharpening process in spatial domain Direct Manipulation of image Pixels. The objective of Sharpening is to highlight transitions in intensity The image blurring is accomplished by pixel averaging in a neighborhood. Since averaging is analogous to integration. Prepared by M. Sahaya Pretha Department of Computer Science and Engineering, MS University, Tirunelveli Dist, Tamilnadu.

Lecture 15 DCT, Walsh and Hadamard TransformVARUN KUMAR

This document discusses discrete cosine, Walsh, and Hadamard transforms for 2D signals. It provides the mathematical formulas for the forward and inverse transforms of each. The discrete cosine transform uses cosine functions in its kernel. The Walsh transform uses the binary representation of values, with the kernel containing terms with (−1) factors. The Hadamard transform has a similar kernel to the Walsh transform. Each transform decomposes 2D signals into component frequencies or patterns in a way that is separable and symmetric.

Image Restoration (Order Statistics Filters)Kalyan Acharjya

Interpixel redundancyNaveen Kumar

The document discusses various techniques for image compression including: - Run-length coding which encodes repeating pixel values and their lengths. - Difference coding which encodes the differences between pixel values. - Block truncation coding which divides images into blocks and assigns codewords. - Predictive coding which predicts pixel values from neighbors and encodes differences. Reversible compression allows exact reconstruction while lossy compression sacrifices some information for higher compression but images remain visually similar. Combining techniques can achieve even higher compression ratios.

Digital Image restorationMd Shabir Alam

Digital image processing involves techniques to restore degraded images. Image restoration aims to recover the original undistorted image from a degraded observation. The degradation is typically modeled as the original image being operated on by a degradation function and additive noise. Common restoration techniques include spatial domain filters like mean, median and order-statistic filters to remove noise, and frequency domain filtering to reduce periodic noise. The choice of restoration method depends on the type and characteristics of degradation in the image.

sodapdf-converzxXxccccCCCCCCCSsted (1).pptxrajukolluri

Popular image restoration techniqueVARUN KUMAR

This document summarizes popular image restoration techniques including inverse filtering, Wiener filtering, and constrained least squares error filtering. It discusses issues with inverse filtering like unsatisfactory results due to noise. Wiener filtering is described as minimizing the mean square error between the original and reconstructed image using the power spectrum of the image and noise. Constrained least squares error filtering estimates images by minimizing noise variance while imposing a smoothness constraint using the Laplacian. Iterative methods for selecting filter parameters like the regularization parameter gamma are also presented.

More Related Content

What's hot (20)

Lecture 16 KL Transform in Image ProcessingVARUN KUMAR

Chapter 5 Image Processing: Fourier TransformationVarun Ojha

Frequency Domain Image Enhancement TechniquesDiwaker Pant

Image Restoration (Digital Image Processing)Kalyan Acharjya

Smoothing Filters in Spatial DomainMadhu Bala

Unit iiChetan Selukar

06 spatial filtering DIPbabak danyal

Image sampling and quantizationBCET, Balasore

Wiener FilterAkshat Ratanpal

Image Filtering in the Frequency DomainAmnaakhaan

Fourier transformNaveen Sihag

Image Enhancement - Point ProcessingGayathri31093

Homomorphic filteringGautam Saxena

Histogram ProcessingAmnaakhaan

Chapter 1 and 2 gonzalez and woodsasodariyabhavesh

Sharpening spatial filtersResearch Scholar in Manonmaniam Sundaranar University

Lecture 15 DCT, Walsh and Hadamard TransformVARUN KUMAR

Image Restoration (Order Statistics Filters)Kalyan Acharjya

Interpixel redundancyNaveen Kumar

Digital Image restorationMd Shabir Alam

Lecture 16 KL Transform in Image ProcessingVARUN KUMAR

Chapter 5 Image Processing: Fourier TransformationVarun Ojha

Frequency Domain Image Enhancement TechniquesDiwaker Pant

Image Restoration (Digital Image Processing)Kalyan Acharjya

Smoothing Filters in Spatial DomainMadhu Bala

Unit iiChetan Selukar

06 spatial filtering DIPbabak danyal

Image sampling and quantizationBCET, Balasore

Wiener FilterAkshat Ratanpal

Image Filtering in the Frequency DomainAmnaakhaan

Fourier transformNaveen Sihag

Image Enhancement - Point ProcessingGayathri31093

Homomorphic filteringGautam Saxena

Histogram ProcessingAmnaakhaan

Chapter 1 and 2 gonzalez and woodsasodariyabhavesh

Sharpening spatial filtersResearch Scholar in Manonmaniam Sundaranar University

Lecture 15 DCT, Walsh and Hadamard TransformVARUN KUMAR

Image Restoration (Order Statistics Filters)Kalyan Acharjya

Interpixel redundancyNaveen Kumar

Digital Image restorationMd Shabir Alam

Similar to Lecture 13 (Usage of Fourier transform in image processing) (20)

sodapdf-converzxXxccccCCCCCCCSsted (1).pptxrajukolluri

Popular image restoration techniqueVARUN KUMAR

Image Restoration (Digital Image Processing)VARUN KUMAR

This document discusses image restoration techniques. It defines image restoration as the process of taking a degraded image and estimating the original clean image. Common types of degradation include motion blur and noise. The document outlines the image formation process and degradation model both in continuous and discrete domains. It describes how degradation can be modeled as a convolution of the original image with a point spread function representing the blurring plus additive noise. The properties of linearity, homogeneity, and position invariance of degradation operators are also covered. Frequency domain techniques and references on image restoration are mentioned.

Lecture 2 Introduction to digital imageVARUN KUMAR

This document provides an introduction to digital images and image processing. It discusses the need for digitization of images, including that digital signals are less affected by noise and interference and can be stored at low cost. It describes the process of digitization, which involves sampling an analog signal to obtain discrete values, and quantizing the signal levels into a finite set of values. Finally, it discusses key aspects of digital images like sampling rate, bandwidth, and reconstructing an image from its sampled and quantized values.

Image Restoration 2 (Digital Image Processing)VARUN KUMAR

This document discusses image restoration techniques. It describes how the degradation model can be estimated through observation of degraded and original images, experimentation to measure the point spread function, or mathematical modeling of the degradation process. It then covers several restoration techniques including inverse filtering, minimum mean squared error filtering, and constrained least squares filtering. These techniques aim to estimate the original true image by inverting the degradation process using knowledge of the point spread function.

Linear Smoothing, Median, and Sharpening FilterVARUN KUMAR

Image trnsformationsJohn Williams

This document discusses frequency domain processing and various image transforms, with a focus on the discrete Fourier transform (DFT). It provides definitions and properties of the DFT, including its relationship to the Fourier transform and examples of applying the DFT to images. Other transforms discussed include the Walsh transform, with examples provided of computing and displaying the Walsh transform of an image. MATLAB code is presented for calculating the DFT and Walsh transform of grayscale images.

Frequency Domain Operation for Image EnhancementVARUN KUMAR

This document discusses frequency domain techniques for image enhancement. It outlines mask processing and frequency domain techniques. It discusses unsharp masking and high boost filtering for sharpening images. It also discusses taking the first order derivative of an image. In the frequency domain, mask processing is equivalent to convolution. A Gaussian function is used as a low pass filter mask in the frequency domain. Examples of low pass and high pass filters are also provided. References for further reading on image processing and computer vision are listed at the end.

Image transformsVisvesvaraya National Institute of Technology, Nagpur, Maharashtra, India

The document discusses digital image processing techniques in the frequency domain. It begins by introducing the discrete Fourier transform (DFT) of one-variable functions and how it relates to sampling a continuous function. It then extends this concept to two-dimensional functions and images. Key topics covered include the 2D DFT and its properties such as translation, rotation, and periodicity. Aliasing in images is also discussed. The document provides examples of how to compute the DFT and inverse DFT of simple images.

DIGITAL IMAGE PROCESSING - Day 4 Image Transformvijayanand Kandaswamy

07 frequency domain DIPbabak danyal

This document provides an overview of frequency domain concepts including the Fourier transform, Fourier series, discrete Fourier transform, and 2D Fourier transform. It discusses how filtering works in the frequency domain by multiplying the Fourier transform of an image with a filter function in the frequency domain before taking the inverse Fourier transform. Filtering in the frequency domain corresponds to convolution in the spatial domain.

Lecture 12 (Image transformation)VARUN KUMAR

This document discusses image transformation, which represents an image as a series of summations of unitary matrices. It describes how 1D signals can be represented as linear combinations of orthogonal basis functions through Fourier analysis. Similarly, images can be transformed and represented as linear combinations of orthonormal basis images using unitary transformations. The transformation decomposes an image into coefficient values that can be used for processing and analysis.

CVD020 - Lecture Week 2Alessandro Palmeri

This document provides an overview and recap of key concepts related to Fourier analysis that are relevant for structural dynamics and earthquake engineering. It defines Fourier series and the Fourier transform, explaining how they can be used to decompose signals into harmonic components in the frequency domain. It also reviews concepts such as the frequency response function and dynamic amplification factor for single-degree-of-freedom oscillators subjected to harmonic loading.

2-D DFT in images.pdfFarah Torkamani-Azar

Frequency Domain Filtering of Digital ImagesUpendra Pratap Singh

Lecture 5 Relationship between pixel-2VARUN KUMAR

The document discusses various topics related to image processing and computer vision, including connected component labeling, distance measures, arithmetic and logical operations, and neighborhood operations on images. Connected component labeling identifies shapes, areas, and boundaries in an image and is a fundamental step for high-level image analysis. Distance measures like Euclidean, city block, and chessboard distance are used to quantify the similarity between pixels. Arithmetic, logical, and neighborhood operations are common image processing techniques used for tasks like noise filtering, edge detection, and thinning.

imagetransforms1-210417050321.pptxMrsSDivyaBME

The document discusses the discrete Fourier transform (DFT) of one- and two-variable functions. It explains that the DFT of a sampled, discrete function can be obtained from the continuous Fourier transform of the underlying sampled function. The DFT provides a way to take samples of the Fourier transform over one period. This is extended to two-dimensional DFTs of digital images. Aliasing effects from under-sampling are described, and properties of the two-dimensional DFT such as periodicity and translation/rotation are covered. Examples of calculating the DFT of simple image matrices are provided.

Image sampling and quantizationBCET, Balasore

1) The document discusses image sampling and quantization in digital images. It defines a digital image as a 2D array of discrete intensity values that are obtained by sampling and quantizing a continuous image. 2) Sampling is described as transforming a continuous signal into discrete values using a sampling interval. This involves multiplying the continuous image by a comb function of delta pulses. 3) Quantization is defined as discretizing the amplitude or intensity values of the sampled image. Together, sampling and quantization allow a continuous image to be represented as a digital image.

Newton's Forward/Backward Difference InterpolationVARUN KUMAR

This document discusses Newton's forward and backward difference interpolation formulas for equally spaced data points. It provides the formulations for calculating the forward and backward differences up to the kth order. For equally spaced points, the forward difference formula approximates a function f(x) using its kth forward difference at the initial point x0. Similarly, the backward difference formula approximates f(x) using its kth backward difference at x0. The document includes an example problem of using these formulas to estimate the Bessel function and exercises involving interpolation of the gamma function and exponential function.

discrete-time-systems and discetre time fourierpranavrajrkmv

sodapdf-converzxXxccccCCCCCCCSsted (1).pptxrajukolluri

Popular image restoration techniqueVARUN KUMAR

Image Restoration (Digital Image Processing)VARUN KUMAR

Lecture 2 Introduction to digital imageVARUN KUMAR

Image Restoration 2 (Digital Image Processing)VARUN KUMAR

Linear Smoothing, Median, and Sharpening FilterVARUN KUMAR

Image trnsformationsJohn Williams

Frequency Domain Operation for Image EnhancementVARUN KUMAR

Image transformsVisvesvaraya National Institute of Technology, Nagpur, Maharashtra, India

DIGITAL IMAGE PROCESSING - Day 4 Image Transformvijayanand Kandaswamy

07 frequency domain DIPbabak danyal

Lecture 12 (Image transformation)VARUN KUMAR

CVD020 - Lecture Week 2Alessandro Palmeri

2-D DFT in images.pdfFarah Torkamani-Azar

Frequency Domain Filtering of Digital ImagesUpendra Pratap Singh

Lecture 5 Relationship between pixel-2VARUN KUMAR

imagetransforms1-210417050321.pptxMrsSDivyaBME

Image sampling and quantizationBCET, Balasore

Newton's Forward/Backward Difference InterpolationVARUN KUMAR

discrete-time-systems and discetre time fourierpranavrajrkmv

More from VARUN KUMAR (20)

Distributed rc ModelVARUN KUMAR

This document discusses and compares lumped RC and distributed RC models. It describes: 1) Lumped RC models treat a wire as a single resistor and capacitor in series, which is inaccurate for long wires. Distributed RC models account for resistance and capacitance per unit length. 2) Distributed RC lines can be modeled by RC trees or RC ladders, where Elmore delay formulas are derived. 3) Delay and time constant in a distributed RC line increase quadratically with wire length, whereas lumped RC models overestimate this relationship. 4) The behavior of a distributed RC line is described by a diffusion equation relating voltage, distance, resistance, and capacitance over time.

Electrical Wire ModelVARUN KUMAR

This document discusses different electrical wire models used to analyze circuit behavior. It begins by introducing lumped models that simplify distributed parasitic elements into single circuit components. A common lumped model is the RC model, which approximates a wire's distributed resistance and capacitance. For long wires, a distributed RC model more accurately captures the wire's continuous resistance and capacitance per unit length. The document concludes by comparing lumped and distributed RC wire models.

Interconnect Parameter in Digital VLSI DesignVARUN KUMAR

This document discusses key interconnect parameters for VLSI design including capacitance, resistance, and inductance. It notes that as device sizes shrink, wire lengths increase which leads to greater parasitic effects that must be considered. The document outlines how capacitance depends on shape and surroundings and can be modeled as parallel plates. Resistance is defined by resistivity, length and cross-sectional area, with aluminum a common interconnect material. Inductance also becomes important at higher frequencies. Models are simplified by ignoring less dominant effects.

Introduction to Digital VLSI DesignVARUN KUMAR

The document introduces digital VLSI design and CMOS technology. It discusses the motivation for digital design, noting advantages like noise immunity and information security. VLSI allows for miniaturization and lower power consumption by increasing storage and speed capabilities. CMOS is introduced as an important ingredient for VLSI design. CMOS combines p-MOS and n-MOS and has low power consumption and noise resistance. It can be used to build inverters, buffers, adders, and other logic gates and chips like microprocessors. The final slide depicts a CMOS inverter.

Challenges of Massive MIMO SystemVARUN KUMAR

This document summarizes a presentation on analyzing massive MIMO systems under different wireless scenarios. It begins with background on mobile communication generations and challenges with exponentially growing data demand. It then discusses massive MIMO as a promising technology for 5G, noting it can support large numbers of users simultaneously and increase spectrum efficiency. However, challenges include hardware mismatch in TDD systems and highly correlated spatial gains. The presentation outlines analyzing the impact of these issues, as well as the feasibility of massive MIMO in cooperative networks. It proposes modeling hardware mismatch and deriving the probability distribution functions of amplitude and phase mismatches. It also discusses using different precoding techniques like zero-forcing to calculate signal-to-interference-plus-noise ratio in the down

E-democracy or Digital DemocracyVARUN KUMAR

The document discusses e-democracy, which uses information and communication technologies to expand and improve democratic processes. E-democracy can enhance democracy by enabling electronic voting, improving civic engagement through online political discussions and information sharing, and allowing more direct participation between citizens and representatives. However, e-democracy systems face issues like ensuring effective citizen participation, voting equality, and addressing cybersecurity risks and protecting sensitive user data. Digital inclusion is also important to ensure all citizens can participate in e-democracy.

Ethics of Parasitic ComputingVARUN KUMAR

This document discusses parasitic computing, which involves getting another program to perform complex computations without its knowledge. Specifically, it can exploit standard internet protocols like TCP and HTTP. Some potential ethical issues are discussed, such as privacy and consent. The conclusion is that an idealist viewpoint may see ethical problems with parasitic computing, while a pragmatist may not, as it relies on normal interactions over the internet that systems implicitly consent to by connecting.

Action Lines of Geneva Plan of ActionVARUN KUMAR

The document outlines the action lines of the Geneva Plan of Action, which includes 5 main points: 1) The role of governments and stakeholders in promoting ICTs, 2) Developing information and communication infrastructure, 3) Increasing access to information and knowledge, 4) Engaging in capacity building, and 5) Building confidence and security in using ICTs. It provides specific recommendations under each point, such as developing national ICT strategies, improving connectivity for schools and libraries, establishing public access points, and supporting research and development.

Geneva Plan of ActionVARUN KUMAR

The Geneva Plan of Action outlines the World Summit on the Information Society (WSIS) process which took place in two phases. The first phase was held in Geneva in 2003 and resulted in a plan of action. The second phase was held in Tunis in 2005 and focused on implementing the Geneva plan and reaching agreements on internet governance and financing mechanisms. Key outcomes included connecting villages, schools, and other institutions to ICTs and ensuring over half the world's population has access to ICTs. UNESCO played a prominent role in both phases and the follow up processes by focusing on themes like education, cultural diversity, and access to information.

Fair Use in the Electronic AgeVARUN KUMAR

This document discusses fair use of copyrighted works in the electronic age. It outlines that individuals, libraries, and educational institutions should be able to make lawful uses of copyrighted works electronically without transaction fees. This includes uses like privately viewing or browsing publicly marketed works, experimenting with variations for fair use purposes, and providing works for study and research. Libraries should also be able to preserve electronic materials and provide them electronically without liability for user actions. Licenses should not restrict fair uses, and public domain works should be freely available electronically for non-profit education.

Software as a PropertyVARUN KUMAR

This document discusses software as property and whether copying proprietary software is wrong. It outlines the history of intellectual property rights, including John Locke's labor theory of property which argues people have a right to what they produce. While software challenges traditional notions of property, most countries consider copying proprietary software without a license to be illegal, though fair use exceptions exist for purposes like criticism, teaching, and research. The act of copying alone may not be wrong philosophically, but using the copied software deprives authors of payment for their labor.

Orthogonal PolynomialVARUN KUMAR

1. The document discusses orthogonal polynomials, which are polynomial sequences where any two different polynomials are orthogonal under some inner product. 2. Some common orthogonal polynomials are Legendre polynomials, Hermite polynomials, Laguerre polynomials, and Chebyshev polynomials. 3. It is proven that for Legendre polynomials pm and pn, the integral from -1 to 1 of pm(x)pn(x)dx is equal to 0 when m is not equal to n, and is equal to 2/(2n+1) when m is equal to n. This shows the orthogonal property of Legendre polynomials.

Patent ProtectionVARUN KUMAR

This document discusses patent protection and its application to software. It begins by recapping trade secrecy law and its limitations for software. It then introduces patents as a stronger form of intellectual property protection that provides a limited-time monopoly on an invention. For software to be patentable, it cannot claim an abstract idea, algorithm, or scientific principle alone; it must demonstrate utility, novelty, and non-obviousness. While software patents were initially controversial, a 1981 court case established that a physical process using a computer program could be patented. The document ends by discussing theories of intellectual property and how software challenges traditional notions of ownership similar to Locke's labor theory.

Copyright Vs Patent and Trade Secrecy Law

This document discusses different mechanisms for intellectual property protection, including copyright, patent, and trade secrecy laws. Copyright protects the expression of ideas but not the ideas themselves. It applies to source and object codes but there are issues around modifications. Trade secrecy laws allow companies to keep information secret to maintain a competitive edge, such as by using non-disclosure agreements. Trade secrecy was more applicable during Bingo's software development but not once the software was released. Patent provides the strongest protection by giving inventors exclusive rights over novel and non-obvious inventions.

Property Right and SoftwareVARUN KUMAR

The document discusses three scenarios related to property rights and software: 1) Ramesh buying pirated software abroad, 2) a small software company called Bingo having their operating system copied, and 3) a man named Jake improving virus detection software and sharing his modifications. It also defines algorithms, source code, and object code. Property rights and copyright issues regarding software are complex with reasonable arguments on both sides.

Investigating Data TrialsVARUN KUMAR

This document discusses different types of data trails that are created when using computers and browsing the internet. It outlines three main types of data trails: 1) those created on your own machine through browser history, cookies, and other files; 2) cookies stored by websites to track user activity; and 3) data trails created on other machines when browsing from work vs home, noting it is easier for employers and internet service providers to track user activity in different ways depending on the connection. The document aims to investigate these data trails without making ethical judgments, simply exploring the purpose and availability of the information collected.

Gaussian Numerical IntegrationVARUN KUMAR

This document discusses Gaussian numerical integration techniques. It describes the Gauss quadrature 2-point and 3-point formulas for numerical integration. The 2-point formula uses two sample points with equal weights of 1 to calculate the integral. The 3-point formula uses three sample points and weights of 5/9, 8/9 and 5/9 to yield more accurate integration over an interval. The document also explains how to apply these formulas when the integral limits differ from [-1,1].

Censorship and ControversyVARUN KUMAR

This document discusses censorship and controversy surrounding it. It outlines strategies for censorship like blocking software and ratings systems. Ratings can be used by closed groups, communities, individuals, or imposed by organizations. There is a tension between freedom of information on the internet and pressure to control or restrict access to certain content, particularly for concerns around inappropriate influence on children or reduced productivity. Education is important to raise awareness of these issues from a global perspective.

Romberg's IntegrationVARUN KUMAR

Romberg's method is used to estimate definite integrals by applying Richardson extrapolation repeatedly to the trapezoidal rule or rectangular rule. This generates a triangular array that increases in accuracy. The method is an extension of trapezoidal and rectangular rules. It works by recursively calculating the integral using smaller step sizes to generate values in the triangular array. Convergence is reached when two successive values are very close. An example calculates a definite integral using Romberg's method in three cases with decreasing step sizes to populate the triangular array.

Introduction to Censorship VARUN KUMAR

Distributed rc ModelVARUN KUMAR

Electrical Wire ModelVARUN KUMAR

Interconnect Parameter in Digital VLSI DesignVARUN KUMAR

Introduction to Digital VLSI DesignVARUN KUMAR

Challenges of Massive MIMO SystemVARUN KUMAR

E-democracy or Digital DemocracyVARUN KUMAR

Ethics of Parasitic ComputingVARUN KUMAR

Action Lines of Geneva Plan of ActionVARUN KUMAR

Geneva Plan of ActionVARUN KUMAR

Fair Use in the Electronic AgeVARUN KUMAR

Software as a PropertyVARUN KUMAR

Orthogonal PolynomialVARUN KUMAR

Patent ProtectionVARUN KUMAR

Property Right and SoftwareVARUN KUMAR

Investigating Data TrialsVARUN KUMAR

Gaussian Numerical IntegrationVARUN KUMAR

Censorship and ControversyVARUN KUMAR

Romberg's IntegrationVARUN KUMAR

Introduction to Censorship VARUN KUMAR

Recently uploaded (20)

theory-slides-for react for beginners.pptxsanchezvanessa7896

Compiler Design_Lexical Analysis phase.pptxRushaliDeshmukh2

ADVXAI IN MALWARE ANALYSIS FRAMEWORK: BALANCING EXPLAINABILITY WITH SECURITYijscai

With the increased use of Artificial Intelligence (AI) in malware analysis there is also an increased need to understand the decisions models make when identifying malicious artifacts. Explainable AI (XAI) becomes the answer to interpreting the decision-making process that AI malware analysis models use to determine malicious benign samples to gain trust that in a production environment, the system is able to catch malware. With any cyber innovation brings a new set of challenges and literature soon came out about XAI as a new attack vector. Adversarial XAI (AdvXAI) is a relatively new concept but with AI applications in many sectors, it is crucial to quickly respond to the attack surface that it creates. This paper seeks to conceptualize a theoretical framework focused on addressing AdvXAI in malware analysis in an effort to balance explainability with security. Following this framework, designing a machine with an AI malware detection and analysis model will ensure that it can effectively analyze malware, explain how it came to its decision, and be built securely to avoid adversarial attacks and manipulations. The framework focuses on choosing malware datasets to train the model, choosing the AI model, choosing an XAI technique, implementing AdvXAI defensive measures, and continually evaluating the model. This framework will significantly contribute to automated malware detection and XAI efforts allowing for secure systems that are resilient to adversarial attacks.

Degree_of_Automation.pdf for Instrumentation and industrial specialistshreyabhosale19

DT REPORT by Tech titan GROUP to introduce the subject design ThinkingDhruvChotaliya2

Artificial Intelligence (AI) basics.pptxaditichinar

Main cotrol jdbjbdcnxbjbjzjjjcjicbjxbcjcxbjcxbSunilSingh610661

Level 1-Safety.pptx Presentation of Electrical SafetyJoseAlbertoCariasDel

Value Stream Mapping Worskshops for Intelligent Continuous SecurityMarc Hornbeek

introduction to machine learining for beginersJoydebSheet

Introduction to Zoomlion Earthmoving.pptxAS1920

Development of MLR, ANN and ANFIS Models for Estimation of PCUs at Different ...Journal of Soft Computing in Civil Engineering

Passenger car unit (PCU) of a vehicle type depends on vehicular characteristics, stream characteristics, roadway characteristics, environmental factors, climate conditions and control conditions. Keeping in view various factors affecting PCU, a model was developed taking a volume to capacity ratio and percentage share of particular vehicle type as independent parameters. A microscopic traffic simulation model VISSIM has been used in present study for generating traffic flow data which some time very difficult to obtain from field survey. A comparison study was carried out with the purpose of verifying when the adaptive neuro-fuzzy inference system (ANFIS), artificial neural network (ANN) and multiple linear regression (MLR) models are appropriate for prediction of PCUs of different vehicle types. From the results observed that ANFIS model estimates were closer to the corresponding simulated PCU values compared to MLR and ANN models. It is concluded that the ANFIS model showed greater potential in predicting PCUs from v/c ratio and proportional share for all type of vehicles whereas MLR and ANN models did not perform well.

How to use nRF24L01 module with ArduinoCircuitDigest

Smart_Storage_Systems_Production_Engineering.pptxrushikeshnavghare94

Raish Khanji GTU 8th sem Internship Report.pdfRaishKhanji

This report details the practical experiences gained during an internship at Indo German Tool Room, Ahmedabad. The internship provided hands-on training in various manufacturing technologies, encompassing both conventional and advanced techniques. Significant emphasis was placed on machining processes, including operation and fundamental understanding of lathe and milling machines. Furthermore, the internship incorporated modern welding technology, notably through the application of an Augmented Reality (AR) simulator, offering a safe and effective environment for skill development. Exposure to industrial automation was achieved through practical exercises in Programmable Logic Controllers (PLCs) using Siemens TIA software and direct operation of industrial robots utilizing teach pendants. The principles and practical aspects of Computer Numerical Control (CNC) technology were also explored. Complementing these manufacturing processes, the internship included extensive application of SolidWorks software for design and modeling tasks. This comprehensive practical training has provided a foundational understanding of key aspects of modern manufacturing and design, enhancing the technical proficiency and readiness for future engineering endeavors.

lecture5.pptxJHKGJFHDGTFGYIUOIUIPIOIPUOHIYGUYFGIHAbodahab

Introduction to FLUID MECHANICS & KINEMATICSnarayanaswamygdas

Fluid mechanics is the branch of physics concerned with the mechanics of fluids (liquids, gases, and plasmas) and the forces on them. Originally applied to water (hydromechanics), it found applications in a wide range of disciplines, including mechanical, aerospace, civil, chemical, and biomedical engineering, as well as geophysics, oceanography, meteorology, astrophysics, and biology. It can be divided into fluid statics, the study of various fluids at rest, and fluid dynamics. Fluid statics, also known as hydrostatics, is the study of fluids at rest, specifically when there's no relative motion between fluid particles. It focuses on the conditions under which fluids are in stable equilibrium and doesn't involve fluid motion. Fluid kinematics is the branch of fluid mechanics that focuses on describing and analyzing the motion of fluids, such as liquids and gases, without considering the forces that cause the motion. It deals with the geometrical and temporal aspects of fluid flow, including velocity and acceleration. Fluid dynamics, on the other hand, considers the forces acting on the fluid. Fluid dynamics is the study of the effect of forces on fluid motion. It is a branch of continuum mechanics, a subject which models matter without using the information that it is made out of atoms; that is, it models matter from a macroscopic viewpoint rather than from microscopic. Fluid mechanics, especially fluid dynamics, is an active field of research, typically mathematically complex. Many problems are partly or wholly unsolved and are best addressed by numerical methods, typically using computers. A modern discipline, called computational fluid dynamics (CFD), is devoted to this approach. Particle image velocimetry, an experimental method for visualizing and analyzing fluid flow, also takes advantage of the highly visual nature of fluid flow. Fundamentally, every fluid mechanical system is assumed to obey the basic laws : Conservation of mass Conservation of energy Conservation of momentum The continuum assumption For example, the assumption that mass is conserved means that for any fixed control volume (for example, a spherical volume)—enclosed by a control surface—the rate of change of the mass contained in that volume is equal to the rate at which mass is passing through the surface from outside to inside, minus the rate at which mass is passing from inside to outside. This can be expressed as an equation in integral form over the control volume. The continuum assumption is an idealization of continuum mechanics under which fluids can be treated as continuous, even though, on a microscopic scale, they are composed of molecules. Under the continuum assumption, macroscopic (observed/measurable) properties such as density, pressure, temperature, and bulk velocity are taken to be well-defined at "infinitesimal" volume elements—small in comparison to the characteristic length scale of the system, but large in comparison to molecular length scale

"Feed Water Heaters in Thermal Power Plants: Types, Working, and Efficiency G...Infopitaara

A feed water heater is a device used in power plants to preheat water before it enters the boiler. It plays a critical role in improving the overall efficiency of the power generation process, especially in thermal power plants. 🔧 Function of a Feed Water Heater: It uses steam extracted from the turbine to preheat the feed water. This reduces the fuel required to convert water into steam in the boiler. It supports Regenerative Rankine Cycle, increasing plant efficiency. 🔍 Types of Feed Water Heaters: Open Feed Water Heater (Direct Contact) Steam and water come into direct contact. Mixing occurs, and heat is transferred directly. Common in low-pressure stages. Closed Feed Water Heater (Surface Type) Steam and water are separated by tubes. Heat is transferred through tube walls. Common in high-pressure systems. ⚙️ Advantages: Improves thermal efficiency. Reduces fuel consumption. Lowers thermal stress on boiler components. Minimizes corrosion by removing dissolved gases.

New Microsoft PowerPoint Presentation.pdfmohamedezzat18803

"Boiler Feed Pump (BFP): Working, Applications, Advantages, and Limitations E...Infopitaara

A Boiler Feed Pump (BFP) is a critical component in thermal power plants. It supplies high-pressure water (feedwater) to the boiler, ensuring continuous steam generation. ⚙️ How a Boiler Feed Pump Works Water Collection: Feedwater is collected from the deaerator or feedwater tank. Pressurization: The pump increases water pressure using multiple impellers/stages in centrifugal types. Discharge to Boiler: Pressurized water is then supplied to the boiler drum or economizer section, depending on design. 🌀 Types of Boiler Feed Pumps Centrifugal Pumps (most common): Multistage for higher pressure. Used in large thermal power stations. Positive Displacement Pumps (less common): For smaller or specific applications. Precise flow control but less efficient for large volumes. 🛠️ Key Operations and Controls Recirculation Line: Protects the pump from overheating at low flow. Throttle Valve: Regulates flow based on boiler demand. Control System: Often automated via DCS/PLC for variable load conditions. Sealing & Cooling Systems: Prevent leakage and maintain pump health. ⚠️ Common BFP Issues Cavitation due to low NPSH (Net Positive Suction Head). Seal or bearing failure. Overheating from improper flow or recirculation.

theory-slides-for react for beginners.pptxsanchezvanessa7896

Compiler Design_Lexical Analysis phase.pptxRushaliDeshmukh2

ADVXAI IN MALWARE ANALYSIS FRAMEWORK: BALANCING EXPLAINABILITY WITH SECURITYijscai

Degree_of_Automation.pdf for Instrumentation and industrial specialistshreyabhosale19

DT REPORT by Tech titan GROUP to introduce the subject design ThinkingDhruvChotaliya2

Artificial Intelligence (AI) basics.pptxaditichinar

Main cotrol jdbjbdcnxbjbjzjjjcjicbjxbcjcxbjcxbSunilSingh610661

Level 1-Safety.pptx Presentation of Electrical SafetyJoseAlbertoCariasDel

Value Stream Mapping Worskshops for Intelligent Continuous SecurityMarc Hornbeek

introduction to machine learining for beginersJoydebSheet

Introduction to Zoomlion Earthmoving.pptxAS1920

Development of MLR, ANN and ANFIS Models for Estimation of PCUs at Different ...Journal of Soft Computing in Civil Engineering

How to use nRF24L01 module with ArduinoCircuitDigest

Smart_Storage_Systems_Production_Engineering.pptxrushikeshnavghare94

Raish Khanji GTU 8th sem Internship Report.pdfRaishKhanji

lecture5.pptxJHKGJFHDGTFGYIUOIUIPIOIPUOHIYGUYFGIHAbodahab

Introduction to FLUID MECHANICS & KINEMATICSnarayanaswamygdas

"Feed Water Heaters in Thermal Power Plants: Types, Working, and Efficiency G...Infopitaara

New Microsoft PowerPoint Presentation.pdfmohamedezzat18803

"Boiler Feed Pump (BFP): Working, Applications, Advantages, and Limitations E...Infopitaara

Lecture 13 (Usage of Fourier transform in image processing)

1. Usage of Fourier Transform in Image Processing Subject: Image Procesing & Computer Vision Dr. Varun Kumar Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 1 / 13

2. Outlines 1 Fourier transform for continuous signal 2 Fourier transform for discrete signal 3 Fast Fourier transform 4 References Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 2 / 13

3. Fourier transform of 1D time varying signal It is a mathematical operation that convert time domain signal to frequency domain signal. Frequency domain signal processing is simpler compare to time domain. Mathematical expression: 1 Fourier transform X(jΩ) = ∞ −∞ x(t)e−jΩt dt CTFT X(ejω ) = ∞ −∞ x(n)e−jωn DTFT (1) 2 Inverse Fourier transform x(t) = 1 2π ∞ −∞ X(jΩ)ejΩt dΩ I−CTFT x(n) = 1 2π ∞ −∞ X(ejω )ejωn I−DTFT (2) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 3 / 13

4. Fourier transform of 1D space varying signal 1 Fourier transform F(f (x)) = F(u) = ∞ −∞ f (x)e−j2πux dx (3) ⇒ Here, f (x) must be continuous and integrable. ⇒ F(u) must be integrable. 2 Inverse Fourier transform F−1 (F(u)) = f (x) = ∞ −∞ F(u)ej2πux du (4) ⇒ F(u) is a complex variable. ⇒ F(u) = FRe(u) + jFIm(u) = |F(u)|ejφ(u) ⇒ Amplitude spectrum : |F(u)| = FRe(u)2 + FIm(u)2 Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 4 / 13

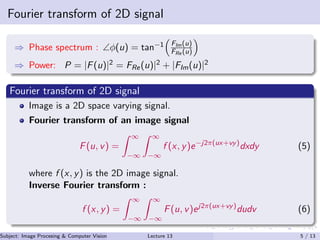
5. Fourier transform of 2D signal ⇒ Phase spectrum : ∠φ(u) = tan−1 FIm(u) FRe (u) ⇒ Power: P = |F(u)|2 = FRe(u)|2 + |FIm(u)|2 Fourier transform of 2D signal Image is a 2D space varying signal. Fourier transform of an image signal F(u, v) = ∞ −∞ ∞ −∞ f (x, y)e−j2π(ux+vy) dxdy (5) where f (x, y) is the 2D image signal. Inverse Fourier transform : f (x, y) = ∞ −∞ ∞ −∞ F(u, v)ej2π(ux+vy) dudv (6) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 5 / 13

6. Continued– ⇒ F(u, v) = |F(u, v)|ejφ(u,v) ⇒ Amplitude spectrum : |F(u, v)| = FRe(u, v)2 + FIm(u, v)2 ⇒ Phase spectrum : φ(u, v) = tan−1 FIm(u,v) FRe (u,v) ⇒ Power spectrum : P(u, v) = |F(u, v)|2 = FRe(u, v)2 + FIm(u, v)2 Example : Find the Fourier transform of a 2D signal which is as follow Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 6 / 13

7. Continued– As per the 2D graphical signal f (x, y) ⇒ f (x, y) = A ∀ 0 ≤ x ≤ X and 0 ≤ y ≤ Y ⇒ f (x, y) = 0 ∀ x > X and y > Y F(u, v) = X 0 Y 0 Ae−j(ux+vy) dxdy = A X 0 e−j2πux dx Y 0 e−j2πvy dy = A 1 j2πu (1 − e−j2πuX ) 1 j2πv (1 − e−j2πvY ) (7) Amplitude spectrum : |F(u, v)| = AXY sin(πuX) πuX sin(πvY ) πvY (8) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 7 / 13

8. Graphical representation 2D discrete signal (DTFT) (Finite dimension) F(u, v) = ∞ −∞ ∞ −∞ f (x, y)e−j2π(ux+vy) (9) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 8 / 13

9. DFT of an image signal DFT/I-DFT of an 2D signal DFT F(u, v) = 1 MN M−1 x=0 N−1 y=0 f (x, y)e−j2π( ux M +vy N ) (10) Here, frequency variable u = 0, 1, ....., M − 1 and v = 0, 1, ...., N − 1 I-DFT f (x, y) = 1 MN M−1 u=0 N−1 v=0 F(u, v)ej2π( ux M +vy N ) (11) In case of square image, i.e, M = N F(u, v) = 1 N N−1 x=0 N−1 y=0 f (x, y)e−j2π( ux N +vy N ) (12) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 9 / 13

10. Properties of Fourier transform 1 Separability: In case of DFT F(u, v) = 1 N N−1 x=0 N−1 y=0 f (x, y)e−j2π(ux N +vy N ) ⇒ 1 N N−1 x=0 e−j 2πux N N. 1 N N−1 y=0 f (x, y)e−j 2πvy N ⇒ 1 N N−1 x=0 NF(x, v)e−j 2πux N (13) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 10 / 13

11. Continued– In case of I-DFT f (x, y) = 1 N N−1 u=0 N−1 v=0 F(u, v)ej2π( ux N +vy N ) ⇒ 1 N N−1 u=0 ej 2πux N N. 1 N N−1 v=0 F(u, v)ej 2πvy N ⇒ 1 N N−1 u=0 Nf (u, y)ej 2πux N (14) 2 Translation f (x, y) ⇒ (x0, y0) ⇒ f (x − x0, y − y0) F(u, v)|x−x0,y−y0 = F(u, v)|x,y e −j2π N (ux0+vy0) (15) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 11 / 13

12. Continued– I-DFT form F(u − u0, v − v0) ⇒ f (x, y)e j2π N (u0x+v0y) (16) Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 12 / 13

13. References M. Sonka, V. Hlavac, and R. Boyle, Image processing, analysis, and machine vision. Cengage Learning, 2014. D. A. Forsyth and J. Ponce, “A modern approach,” Computer vision: a modern approach, vol. 17, pp. 21–48, 2003. L. Shapiro and G. Stockman, “Computer vision prentice hall,” Inc., New Jersey, 2001. R. C. Gonzalez, R. E. Woods, and S. L. Eddins, Digital image processing using MATLAB. Pearson Education India, 2004. Subject: Image Procesing & Computer Vision Dr. Varun Kumar (IIIT Surat)Lecture 13 13 / 13

Lecture 13 (Usage of Fourier transform in image processing)

Recommended

More Related Content

What's hot (20)

Similar to Lecture 13 (Usage of Fourier transform in image processing) (20)

More from VARUN KUMAR (20)

Recently uploaded (20)

Lecture 13 (Usage of Fourier transform in image processing)