Statistics and Probability-Random Variables and Probability Distribution

Random Variable
-assigns a number to each
outcome of a random
circumstance, or,
equivalently, to each unit in
a population.
-is a number generated by a
random experiment

Two different types of random variables:
*1. A continuous random variable can take
any value in an interval or collection of
intervals. Its possible values contain a
whole interval of numbers.
*2. A discrete random variable can take one
of a countable list of distinct values. Its
possible values form a finite or countable
set.
*Notation for either type: X, Y, Z, W, etc.

Examples of Discrete Random Variables Assigns a
number to each outcome in the sample space for a
random circumstance, or to each unit in a
population.
1. Couple plans to have 3 children. The random
circumstance includes the 3 births, specifically
the sexes of the 3 children. Possible outcomes
(sample space): BBB, BBG, etc.
X = number of girls
X is discrete and can be 0, 1, 2, 3
For example, the number assigned to BBB is X=0
2. Population consists of students (unit = student)
Y = number of siblings a student has
Y is discrete and can be 0, 1, 2, …??

Examples of Continuous Random Variables
Assigns a number to each outcome of a
random circumstance, or to each unit in a
population.
1.Population consists of female students
Unit = female student
W = height
W is continuous – can be anything in an
interval, even if we report it to nearest inch or
half inch
2. You are waiting at a bus stop for the next bus
Random circumstance = when the bus arrives
Y = time you have to wait
Y is continuous – anything in an interval

2 TYPES OF RANDOM VARIABLES
DISCRETE RANDOM VARIABLES
Number of scales
Number of calls
People in a line
Score in an exam
CONTINUOUS RANDOM VARIABLES
Length
Depth
Volume
Time
Weight

EXPERIMENT RANDOM VARIABLE POSSIBLE VALUES
of X
Roll two fair dice X=Sum of the
number of dots on
the top face
2, 3, 4, 5, 6, 7, 8,
9, 10, 11, 12
Flip a fair coin
repeatedly
X=Number of
tosses until the
coin lands heads
1, 2, 3, 4, …
Measure of voltage
at an electrical
outlet
X=Voltage
measured
118<x<122
The air pressure of
a tire on an
automobile
X=Air pressure 30<x<32

Classify each random variable as either
discrete or continuous.
1.The number of arrivals a an emergency
room between midnight and 6:00 a.m.
2.The weight of a box of cereal
3.The duration of the next outgoing call
from a business office
4.The number of boys in a randomly
selected thee-child policy
5.The temperature of a cup of coffee
served at a restaurant.

Classify each random variable as either
discrete or continuous.
1. The number of applicants in a job
2. The time between customers
entering a checkout lane at a retail
store.
3. The average amount of electricity a
household consume in a month
4. The number of accident-free days
in one month at a factory
5. The number of vehicles owned by a
government official

The number of heads in two tosses
of a coin
The average weight of newborn
babies in the Philippines
The number of games in the
basketball boys of junior high
The number of coins that match
when three coins are tossed at once
Identify the set of possible values for each
random variable.

Learning Competency:
Illustrates a probability distribution
for a discrete random variable and its
properties
*

What is the probability of getting
a head in a toss of a coin
What is the pro babilityof getting
a Queen of Heart ♥ in a deck of
cards?
What is the probability of a
female Grade 11 Stem Student to
be chosen from their section?

Probability Distributions
Of a discrete random
variable X is a list of each
possible value of X
together with the
probability that X takes
that value in one trial of
the experiment

The probabilities in the probability distribution of a
random variable X must satisfy the following two
conditions:
1. Each probability P (x) must be
between 0 and 1:
0 ≤ P (x) ≤ 1.
2. The sum of all the probabilities is 1:
ΣP(x) = 1.

EXAMPLE 1
A fair coin is tossed twice. Let X be the number of heads
that are observed.
a. Construct the probability distribution of X.
b. Find the probability that at least one head is observed.
Solution:
a. The possible values that X can take are 0, 1, and 2. Each of
these numbers corresponds to an event in the sample space
S = {hh, ht, th, tt} of equally likely outcomes for this
experiment: X = 0 to {tt}, X = 1 to {ht, th} , and X = 2 to
{hh}. The probability of each of these events, hence of the
corresponding value of X, can be found simply by counting,
to give x 0 1 2
P(x) ¼ or 0.25 2/4 or 0.50 ¼ or 0.25
This table is the probability distribution of X.

A histogram that graphically
illustrates the probability
distribution is given in Figure 4.1
"Probability Distribution for
Tossing a Fair Coin Twice".

EXAMPLE 2
A pair of fair dice is rolled. Let X denote the sum of the
number of dots on the top faces.
a. Construct the probability distribution of X.
b. Find P(X ≥ 9).
c. Find the probability that X takes an even value.
Solution:
The sample space of equally likely outcomes is
11 12 13 14 15 16
21 22 23 24 25 26
31 32 33 34 35 36
41 42 43 44 45 46
51 52 53 54 55 56
61 62 63 64 65 66

This table is the probability distribution of X.
a. The possible values for X are the numbers 2 through 12.
X = 2 is the event {11}, so P (2) =
1
36
.
X = 3 is the event {12,21}, so P (3) =
2
36
.
Continuing this way we obtain the table
x 2 3 4 5 6 7 8 9 10 11 12
P(x) 1
36
2
36
3
36
4
36
5
36
6
36
5
36
4
36
3
36
2
36
1
36

b. The event X ≥ 9 is the union of the mutually exclusive
events X =9, X = 10, X = 11, and X = 12. Thus
P (X ≥ 9) = P(9) +P(10) +P(11) +P(12)
=
𝟒
𝟑𝟔
+
𝟑
𝟑𝟔
+
𝟐
𝟑𝟔
+
𝟏
𝟑𝟔
=
𝟓
𝟏𝟖
c. Note that X takes six different even values but only five
different odd values. We compute
P(X is even)=P(2)+ P(4)+ P(6)+ P(8)+ P(10)+ P(12)
=
𝟏
𝟑𝟔
+
𝟑
𝟑𝟔
+
𝟓
𝟑𝟔
+
𝟓
𝟑𝟔
+
𝟑
𝟑𝟔
+
𝟏
𝟑𝟔
=
𝟏𝟖
𝟑𝟔
or 0.5
x 2 3 4 5 6 7 8 9 10 11 12
P(x) 1
36
2
36
3
36
4
36
5
36
6
36
5
36
4
36
3
36
2
36
1
36

A histogram that graphically
illustrates the probability
distribution is given in Figure 4.2
"Probability Distribution for
Tossing Two Fair Dice".

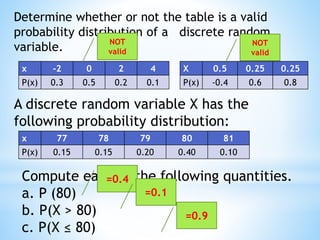
Determine whether or not the table is a valid
probability distribution of a discrete random
variable.
x -2 0 2 4
P(x) 0.3 0.5 0.2 0.1
X 0.5 0.25 0.25
P(x) -0.4 0.6 0.8
A discrete random variable X has the
following probability distribution:
x 77 78 79 80 81
P(x) 0.15 0.15 0.20 0.40 0.10
Compute each of the following quantities.
a. P (80)
b. P(X > 80)
c. P(X ≤ 80)

Determine whether or not the table is a valid
probability distribution of a discrete random
variable.
x -2 0 2 4
P(x) 0.3 0.5 0.2 0.1
X 0.5 0.25 0.25
P(x) -0.4 0.6 0.8
x 77 78 79 80 81
P(x) 0.15 0.15 0.20 0.40 0.10
a. P (80)
b. P(X > 80)
c. P(X ≤ 80)
NOT
valid
NOT
valid
=0.4
=0.1
=0.9

Learning Competency:
Illustrates the mean, variance &
standard deviation of a discrete random
variable
Calculates the mean, variance &
standard deviation of a discrete random
variable
*

Definition
The mean (also called the
expected value) of a discrete random
variable X is the number
μ = Σ [x·P(x)]
The mean of a random variable may be
interpreted as the average of the
values assumed by the random variable
in repeated trials of the experiment.

EXAMPLE
Find the mean of the discrete random
variable X whose probability
distribution is
x -2 1 2 3.5
P(x) 0.21 0.34 0.24 0.21
Solution:
The formula in the definition gives
μ =Σx P(x)
=(−2) · 0.21 + (1) · 0.34 + (2) · 0.24 +(3.5)· 0.21
= 1.135

Definition
The variance, σ2, of a discrete
random variable X is the
number σ2= Σ(x − μ)2P(x)
which by algebra is equivalent
to the formula
σ2=[Σ x2P(x)]−μ2

Definition
The standard deviation , σ, of a discrete
random variable X is the square root of its
variance, hence is given by the formula
σ = 𝝈 𝟐
The variance and standard deviation of a
discrete random variable X may be
interpreted as measures of the variability of
the values assumed by the random variable
in repeated trials of the experiment. The
units on the standard deviation match those
of X.

EXAMPLE
Find the variance and standard deviation
of the discrete random variable X whose
probability distribution is
X -1 0 1 4
P(x) 0.2 0.5 0.2 0.1
a. The mean μ of X.
b. The variance σ2 of X.
c. The standard deviation σ of X.

Solution:
a.Using the formula in the definition of μ,
μ = Σx P (x)
= (−1) · 0.2 + 0 · 0.5 + 1 · 0.2 + 4 · 0.1
= 0.4
b. Using the formula in the definition of σ2 and
the value of μ that was just computed,
σ2=[Σ x2P(x)]−μ2
= [(−1)2 ·0.2 + 02 ·0.5 + 12 ·0.2 + 42 ·0.1]-0.42
=1.84
c. Using the result of b,
σ = 1.84
= 1.3565

x 77 78 79 80 81
P(x) 0.15 0.15 0.20 0.40 0.10
f. The standard deviation σ of X.

x 77 78 79 80 81
P(x) 0.15 0.15 0.20 0.40 0.10
a. The mean μ of X. ans.=79.15
b. The variance σ2 of X. ans.=1.5275
f. The standard deviation σ of X. ans.=1.24

Two fair dice are rolled
at once. Let X denote
the difference in the
number of dots that
appear on the top faces
of the two dice. Thus for
example if a one and a
five are rolled, X = 4,
and if two sixes are
rolled, X = 0.
a. Construct the
probability
distribution for X.
b. Compute the
mean μ of X.
c. Compute the
standard deviation σ
of X.

DIFFERENCE OF TWO DOTS
0 1 2 3 4 5
1 0 1 2 3 4
2 1 0 1 2 3
3 2 1 0 1 2
4 3 2 1 0 1
5 4 3 2 1 0
0 6
X 0 1 2 3 4 5
1 10
P(X) 1/6 5/18 2/9 1/6 1/9 1/18 1
2 8
3 6
4 4
5 2
TOTAL 36

DIFFERENCE OF TWO DOTS
0 1 2 3 4 5
1 0 1 2 3 4
2 1 0 1 2 3
3 2 1 0 1 2
4 3 2 1 0 1
5 4 3 2 1 0
0 6
X 0 1 2 3 4 5
1 10
P(X) 1/6 5/18 2/9 1/6 1/9 1/18 1
2 8
3 6
MEAN 1.94
4 4
VARIANCE 2.07
5 2
SD 1.44
TOTAL 36

TEST I. Solve the following:
1. A grocery store has determined that
in crates of tomatoes, 95% carry no
rotten tomatoes, 2% carry one rotten
tomato, 2% carry two rotten tomatoes,
and 1% carry three rotten tomatoes.
a. Find the mean number of rotten tomatoes in the
crates.”
b. What is P(x>1)?

2. Probability distribution
that results from the rolling
of a single fair die.
x 1 2 3 4 5 6
p(x) 1/6 1/6 1/6 1/6 1/6 1/6
Compute each of the following
quantities.
c. The standard deviation σ of X.

3. Suppose an individual plays a gambling
game where it is possible to lose $1.00,
break even, win $3.00, or win $10.00
each time she plays. The probability
distribution for each outcome is provided
by the following table:
Outcome -$1.00 $0.00 $3.00 $5.00
Probability 0.30 0.40 0.20 0.10
a. Find the mean
b. Find the variance.

4. Let X denote the number of boys
in a randomly selected three-child
family. Assuming that boys and girls
are equally likely, construct the
probability distribution of X.
5. Let X denote the number of times
a fair coin lands heads in three
tosses. Construct the probability
distribution of X.

TEST II. Identify the following
variables(DISCRETE OR CONTINUOUS):
1. distance traveled between classes
2. number of students present
3. height of students in class
4. number of red marbles in a jar
5. time it takes to get to school
6. number of heads when flipping three coins
7. students’ grade level
8. The temperature of a cup of coffee served
at a restaurant
9. The number of applicants for a job.
10.weight of students in class

TEST III. Determine whether or not the table is a
valid probability distribution of a discrete
random variable.
1.
2.
3.
4.
5.
x -2 0 2 4
P(x) 0.4 0.5 0.1 0.1
X 0.5 0.25 0.25
P(x) 0.2 0.6 0.2
x 5 6 7 8
P(x) -0.1 0.5 0.4 0.2
X -4 -3 -2 -1
P(x) 0.25 0.20 0.40 0.15
X 1 2 3 4
P(x) ¼ ¼ ¼ ¼

1. a. 0.09 b. 0.03
2. a. 7/2 b. 2.92
c. 1.71
3. a. 0.8b. 3.36
c. 1.83

1. Let X denote the number of times
a fair coin lands heads in three
tosses. Construct the probability
distribution of X.

Statistics and Probability-Random Variables and Probability Distribution

Recommended

More Related Content

What's hot (20)

Similar to Statistics and Probability-Random Variables and Probability Distribution (20)

Recently uploaded (20)

Statistics and Probability-Random Variables and Probability Distribution